Wiener equation: Difference between revisions
en>Melcombe revise cats, revise stub templarte |
en>EmausBot m Bot: Migrating 1 interwiki links, now provided by Wikidata on d:Q12346869 |
||
| Line 1: | Line 1: | ||
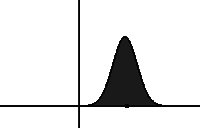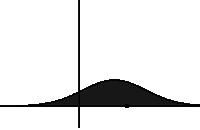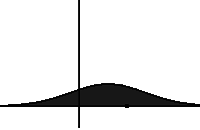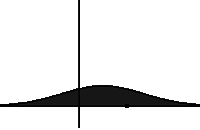
[[Image:FokkerPlanck.gif|thumb|A solution to the one-dimensional Fokker–Planck equation, with both the drift and the diffusion term. In this case the initial condition is a [[Dirac delta function]] centered away from zero velocity. Over time the distribution widens due to random impulses, and relaxes towards zero velocity due to drag.]] | |||
In [[statistical mechanics]], the '''Fokker–Planck equation''' is a [[partial differential equation]] that describes the [[time evolution]] of the [[probability density function]] of the velocity of a particle under the influence of drag forces and random forces, as in [[Brownian motion]]. The equation can be generalized to other observables as well.<ref>{{Cite book| title = Statistical Physics: statics, dynamics and renormalization| author = Leo P. Kadanoff| publisher = World Scientific| isbn = 981-02-3764-2| year = 2000| url = http://books.google.com/?id=22dadF5p6gYC&pg=PA135&dq=Fokker%E2%80%93Planck}}</ref> | |||
It is named after [[Adriaan Fokker]]<ref>A. D. Fokker, | |||
''Die mittlere Energie rotierender elektrischer Dipole im Strahlungsfeld'', | |||
Ann. Phys. 348 (4. Folge 43), 810–820 (1914).</ref> | |||
and [[Max Planck]]<ref> | |||
M. Planck, Sitz.ber. Preuß. Akad. (1917).</ref> | |||
and is also known as the '''Kolmogorov forward equation''' (diffusion), named after [[Andrey Kolmogorov]], who first introduced it in a 1931 paper.<ref>Andrei Kolmogorov, "On Analytical Methods in the Theory of Probability", 448-451, (1931), (in German).</ref> | |||
When applied to particle position distributions, it is better known as the [[Smoluchowski equation]]. The case with zero diffusion is known in statistical mechanics as [[Liouville's theorem (Hamiltonian)|Liouville equation]]. | |||
The first consistent microscopic derivation of the Fokker–Planck equation in the single scheme of classical and quantum mechanics was performed<ref>[[Nikolay Boglyubov (jr)|N. N. Bogolyubov (jr)]] and D. P. Sankovich (1994). "[http://dx.doi.org/10.1070/RM1994v049n05ABEH002419 N. N. Bogolyubov and statistical mechanics]". ''Russian Math. Surveys'' '''49'''(5): 19—49.</ref> | |||
by [[Nikolay Bogoliubov]] and [[Nikolay Mitrofanovich Krylov|Nikolay Krylov]].<ref>[[Nikolay Bogoliubov|N. N. Bogoliubov]] and [[Nikolay Mitrofanovich Krylov|N. M. Krylov]] (1939). ''Fokker–Planck equations generated in perturbation theory by a method based on the spectral properties of a perturbed Hamiltonian''. Zapiski Kafedry Fiziki Akademii Nauk Ukrainian SSR '''4''': 81–157 (in Ukrainian).</ref> | |||
== One dimension == | |||
In one spatial dimension ''x'', for an [[Itō calculus|Itō]] process given by the [[stochastic differential equation]] | |||
:<math>dX_t = \mu(X_t,t)dt + \sqrt{2 D(X_t,t)}dW_t</math> | |||
with drift <math>\mu(X_t,t)</math> and [[diffusion]] coefficient <math>D(X_t,t)</math>, the Fokker–Planck equation for the probability density <math>f(x,t)</math> of the random variable <math>X_t</math> is | |||
:<math>\frac{\partial}{\partial t}f(x,t) = -\frac{\partial}{\partial x}\left[\mu(x,t)f(x,t)\right] + \frac{\partial^2}{\partial x^2}\left[ D(x,t)f(x,t)\right].</math> | |||
The link between stochastic differential equations and partial differential equations is given by the [[Feynman-Kac formula]]. | |||
The stochastic process defined above in the Ito sense can be rewritten within the [[Stratonovich integral|Stratonovich]] convention as a Stratonovich SDE: | |||
:<math>dX_t = \left[\mu(X_t,t) - \frac{1}{2} \frac{\partial}{\partial X_t}D(X_t,t)\right]dt + \sqrt{2 D(X_t,t)} \circ dW_t.</math> | |||
It includes an added noise-induced drift term due to diffusion gradient effects if the noise is state-dependent. This convention is more often used in physical applications. Indeed, it is well known that any solution to the Stratonovich SDE is a solution to the Ito SDE. | |||
== Many dimensions == | |||
More generally, if <math>\mathbf{X}_t</math> is an ''N''-dimensional random [[vector (geometry)|vector]] and <math>\mathbf{W}_t</math> is an ''M''-dimensional standard [[Wiener process]], | |||
:<math>d\mathbf{X}_t = \boldsymbol{\mu}(\mathbf{X}_t,t)\,dt + \boldsymbol{\sigma}(\mathbf{X}_t,t)\,d\mathbf{W}_t,</math> | |||
the probability density <math>f(\mathbf{x},t)</math> for the random vector <math>\mathbf{X}_t</math> satisfies the Fokker–Planck equation | |||
:<math>\frac{\partial f(\mathbf{x},t)}{\partial t} = -\sum_{i=1}^N \frac{\partial}{\partial x_i} \left[ \mu_i(\mathbf{x}) f(\mathbf{x},t) \right] + \sum_{i=1}^{N} \sum_{j=1}^{N} \frac{\partial^2}{\partial x_i \, \partial x_j} \left[ D_{ij}(\mathbf{x}) f(\mathbf{x},t) \right],</math> | |||
with drift vector <math>\boldsymbol{\mu} = (\mu_1,\ldots,\mu_N)</math> and diffusion [[tensor]] | |||
:<math>D_{ij}(\mathbf{x},t) = \frac{1}{2} \sum_{k=1}^M \sigma_{ik}(\mathbf{x},t) \sigma_{jk}(\mathbf{x},t).</math> | |||
== Examples == | |||
A standard scalar [[Wiener process]] is generated by the [[stochastic differential equation]] | |||
:<math>dX_t = dW_t.</math> | |||
Here the drift term is zero and the diffusion coefficient is 1/2. Thus the corresponding Fokker–Planck equation is | |||
:<math> | |||
\frac{\partial f(x,t)}{\partial t} = \frac{1}{2} \frac{\partial^2 f(x,t)}{\partial x^2}, | |||
</math> | |||
which is the simplest form of a [[diffusion equation]]. If the initial condition is <math>f(x,0) = \delta(x)</math>, the solution is | |||
:<math>f(x,t) = \frac{1}{\sqrt{2 \pi t}}e^{-{x^2}/({2t})}.</math> | |||
Alternatively, in plasma physics, the [[distribution function]] for a particle species <math>s</math>, <math>f_{s} \left(\vec{x},\vec{v},t\right)</math>, takes the place of the [[probability density function]]. The corresponding Fokker-Planck equation is given by | |||
<math>\frac{\partial f_{s}}{\partial t} + \vec{v} \cdot \vec{\nabla} f_{s} + \frac{Z_{s} e}{m_{s}} \left( \vec{E} + \vec{v} \times \vec{B} \right) \cdot \vec{\nabla}_{v} f_{s} = \sum_{s'} C\left[f_{s},f_{s'}\right]</math>, | |||
where the third term includes the particle acceleration due to the [[Lorentz force]] and the right-hand side represents the effects of particle collisions. If collisions are ignored the Fokker-Planck equation reduces to the [[Vlasov equation]]. | |||
==Computational considerations== | |||
Brownian motion follows the [[Langevin equation]], which can be solved for many different stochastic forcings with results being averaged (the [[Monte Carlo method]], canonical ensemble in [[molecular dynamics]]). However, instead of this computationally intensive approach, one can use the Fokker–Planck equation and consider the probability <math>f(\mathbf{v}, t)d\mathbf{v}</math> of the particle having a velocity in the interval <math>(\mathbf{v}, \mathbf{v} + d\mathbf{v})</math> when it starts its motion with <math>\mathbf{v}_0</math> at time 0. | |||
==Solution== | |||
Being a [[partial differential equation]], the Fokker–Planck equation can be solved analytically only in special cases. A formal analogy of the Fokker–Planck equation with the [[Schrödinger equation]] allows the use of advanced operator techniques known from quantum mechanics for its solution in a number of cases. | |||
In many applications, one is only interested in the steady-state probability distribution | |||
<math> f_0(x)</math>, which can be found from <math>\dot{f}_0(x)=0</math>. | |||
The computation of mean [[first passage time]]s and splitting probabilities can be reduced to the solution of an ordinary differential equation which is intimately related to the Fokker–Planck equation. | |||
==Particular cases with known solution and inversion== | |||
In [[mathematical finance]] for [[volatility smile]] modeling of options via [[local volatility]], one has the problem of deriving a diffusion coefficient <math>{\sigma}(\mathbf{X}_t,t)</math> consistent with a probability density obtained from market option quotes. The problem is therefore an inversion of the Fokker Planck–equation: Given the density f(x,t) of the option underlying X deduced from the option market, one aims at finding the local volatility <math>{\sigma}(\mathbf{X}_t,t)</math> consistent with f. This is an [[inverse problem]] that has been solved in general by Dupire (1994, 1997) with a non-parametric solution. Brigo and Mercurio (2002, 2003) propose a solution in parametric form via a particular local volatility <math>{\sigma}(\mathbf{X}_t,t)</math> consistent with a solution of the Fokker–Planck equation given by a [[mixture model]]. More information is available also in Fengler (2008), Gatheral (2008) and Musiela and Rutkowski (2008). | |||
==Fokker–Planck equation and path integral== | |||
Every Fokker–Planck equation is equivalent to a [[Path Integral Formulation|path integral]]. The path integral formulation is an excellent starting point for the application of field theory methods.<ref>{{Cite book|author=Zinn-Justin, Jean |title=Quantum field theory and critical phenomena |publisher=Clarendon Press |location=Oxford |year=1996 |pages= |isbn=0-19-851882-X |oclc= |doi= |accessdate=}}</ref> This is used, for instance, in [[Critical_phenomena#Critical_dynamics|critical dynamics]]. | |||
A derivation of the path integral is possible in the same way as in quantum mechanics, simply because the Fokker–Planck equation is formally equivalent to the [[Schrödinger equation]]. Here are the steps for a Fokker–Planck equation with one variable x. | |||
Write the FP equation in the form | |||
:<math>\frac{\partial }{\partial t}f\left( x^{\prime },t\right) =\int_{-\infty}^\infty dx\left( \left[ D_{1}\left( x,t\right) \frac{\partial }{\partial x}+D_2 \left( x,t\right) \frac{\partial^2}{\partial x^2}\right] \delta\left( x^{\prime }-x\right) \right) f\left( x,t\right).</math> | |||
The x-derivatives here only act on the <math>\delta</math>-function, not on <math>f(x,t)</math>. Integrate over a time interval <math>\varepsilon</math>, | |||
:<math>f\left( x^\prime ,t+\varepsilon \right) =\int_{-\infty }^\infty \, dx\left(\left( 1+\varepsilon \left[ D_{1}\left(x,t\right) \frac{\partial }{\partial x}+D_{2}\left( x,t\right) \frac{\partial ^{2}}{\partial x^{2}}\right]\right) \delta \left( x^\prime - x\right) \right) f\left( x,t\right)+O\left( \varepsilon ^{2}\right).</math> | |||
Insert the [[Fourier integral]] | |||
:<math>\delta \left( x^{\prime }-x\right) =\int_{-i\infty }^{i\infty} \frac{d \tilde{x}}{2\pi i }e^{\tilde{x}\left( x-x^{\prime}\right)}</math> | |||
for the <math>\delta</math>-function, | |||
:<math> | |||
\begin{align} | |||
f\left( x^{\prime },t+\varepsilon \right) & = \int_{-\infty }^\infty dx\int_{-i\infty }^{i\infty } \frac{d\tilde{x}}{2\pi i} \left(1+\varepsilon \left[ \tilde{x}D_{1}\left( x,t\right) +\tilde{x}^{2}D_{2}\left( x,t\right) \right] \right) e^{\tilde{x}\left(x-x^{\prime }\right) }f\left( x,t\right) +O\left( \varepsilon ^{2}\right) \\ | |||
& =\int_{-\infty }^\infty dx\int_{-i\infty }^{i\infty }\frac{d\tilde{x}}{2\pi i}\exp \left( \varepsilon \left[ -\tilde{x}\frac{\left( x^{\prime}-x\right) }{\varepsilon }+\tilde{x}D_{1}\left( x,t\right) +\tilde{x}^{2}D_{2}\left( x,t\right) \right] \right) f\left( x,t\right) +O\left(\varepsilon ^{2}\right). | |||
\end{align} | |||
</math> | |||
This equation expresses <math>f\left( x^\prime ,t+\varepsilon \right)</math> as functional of <math>f\left( x,t\right)</math>. Iterating <math>\left( t^\prime -t\right)/\varepsilon</math> times and performing the limit <math>\varepsilon \longrightarrow 0</math> gives a [[Path Integral Formulation|path integral]] with [[Lagrangian]] | |||
:<math>L=\int dt\left[ \tilde{x}D_1 \left( x,t\right) +\tilde{x}^{2}D_2 \left( x,t\right) -\tilde{x}\frac{\partial x}{\partial t}\right].</math> | |||
The variables <math>\tilde{x}</math> conjugate to <math>x</math> are called "response variables".<ref name="Janssen">{{Cite journal|last=Janssen |first=H. K. |title=On a Lagrangean for Classical Field Dynamics and Renormalization Group Calculation of Dynamical Critical Properties |journal=Z. Physik |volume=B23 |issue= 4|pages=377–380 |year=1976 |doi=10.1007/BF01316547|bibcode = 1976ZPhyB..23..377J }}</ref> | |||
Although formally equivalent, different problems may be solved more easily in the Fokker–Planck equation or the path integral formulation. The equilibrium distribution for instance may be obtained more directly from the Fokker–Planck equation. | |||
==See also== | |||
*[[Kolmogorov backward equations (diffusion)|Kolmogorov backward equation]] | |||
*[[Boltzmann equation]] | |||
*[[Navier–Stokes equations]] | |||
*[[Vlasov equation]] | |||
*[[Master equation]] | |||
*[[BBGKY hierarchy|Bogoliubov–Born–Green–Kirkwood–Yvon hierarchy of equations]] | |||
*[[Ornstein–Uhlenbeck process]] | |||
*[[Cointelation]] SDE | |||
==Notes and references== | |||
{{Reflist}} | |||
==Further reading== | |||
*[[Bruno Dupire]] (1994) Pricing with a Smile. Risk Magazine, January, 18–20. | |||
*[[Bruno Dupire]] (1997) Pricing and Hedging with Smiles. Mathematics of Derivative Securities. Edited by M.A.H. Dempster and S.R. Pliska, Cambridge University Press, Cambridge, 103–111. ISBN 0-521-58424-8. | |||
*{{Cite doi|10.1142/S0219024902001511}} | |||
*{{Cite doi|10.1088/1469-7688/3/3/303}} | |||
*Fengler, M. R. (2008). Semiparametric Modeling of Implied Volatility, 2005, Springer Verlag, ISBN 978-3-540-26234-3 | |||
* Crispin Gardiner (2009), "Stochastic Methods", 4th edition, Springer, ISBN 978-3-540-70712-7. | |||
*[[Jim Gatheral]] (2008). The Volatility Surface. Wiley and Sons, ISBN 978-0-471-79251-2. | |||
*Marek Musiela, Marek Rutkowski. Martingale Methods in Financial Modelling, 2008, 2nd Edition, Springer-Verlag, ISBN 978-3-540-20966-9. | |||
* Hannes Risken, "The Fokker–Planck Equation: Methods of Solutions and Applications", 2nd edition, Springer Series in Synergetics, Springer, ISBN 3-540-61530-X. | |||
==External links== | |||
*[http://jeff560.tripod.com/f.html Fokker–Planck equation] on the [http://jeff560.tripod.com/mathword.html Earliest known uses of some of the words of mathematics] | |||
{{DEFAULTSORT:Fokker-Planck Equation}} | |||
[[Category:Stochastic processes]] | |||
[[Category:Equations]] | |||
[[Category:Parabolic partial differential equations]] | |||
Latest revision as of 01:56, 12 May 2013
In statistical mechanics, the Fokker–Planck equation is a partial differential equation that describes the time evolution of the probability density function of the velocity of a particle under the influence of drag forces and random forces, as in Brownian motion. The equation can be generalized to other observables as well.[1] It is named after Adriaan Fokker[2] and Max Planck[3] and is also known as the Kolmogorov forward equation (diffusion), named after Andrey Kolmogorov, who first introduced it in a 1931 paper.[4] When applied to particle position distributions, it is better known as the Smoluchowski equation. The case with zero diffusion is known in statistical mechanics as Liouville equation.
The first consistent microscopic derivation of the Fokker–Planck equation in the single scheme of classical and quantum mechanics was performed[5] by Nikolay Bogoliubov and Nikolay Krylov.[6]
One dimension
In one spatial dimension x, for an Itō process given by the stochastic differential equation
with drift and diffusion coefficient , the Fokker–Planck equation for the probability density of the random variable is
The link between stochastic differential equations and partial differential equations is given by the Feynman-Kac formula.
The stochastic process defined above in the Ito sense can be rewritten within the Stratonovich convention as a Stratonovich SDE:
It includes an added noise-induced drift term due to diffusion gradient effects if the noise is state-dependent. This convention is more often used in physical applications. Indeed, it is well known that any solution to the Stratonovich SDE is a solution to the Ito SDE.
Many dimensions
More generally, if is an N-dimensional random vector and is an M-dimensional standard Wiener process,
the probability density for the random vector satisfies the Fokker–Planck equation
with drift vector and diffusion tensor
Examples
A standard scalar Wiener process is generated by the stochastic differential equation
Here the drift term is zero and the diffusion coefficient is 1/2. Thus the corresponding Fokker–Planck equation is
which is the simplest form of a diffusion equation. If the initial condition is , the solution is
Alternatively, in plasma physics, the distribution function for a particle species , , takes the place of the probability density function. The corresponding Fokker-Planck equation is given by
where the third term includes the particle acceleration due to the Lorentz force and the right-hand side represents the effects of particle collisions. If collisions are ignored the Fokker-Planck equation reduces to the Vlasov equation.
Computational considerations
Brownian motion follows the Langevin equation, which can be solved for many different stochastic forcings with results being averaged (the Monte Carlo method, canonical ensemble in molecular dynamics). However, instead of this computationally intensive approach, one can use the Fokker–Planck equation and consider the probability of the particle having a velocity in the interval when it starts its motion with at time 0.
Solution
Being a partial differential equation, the Fokker–Planck equation can be solved analytically only in special cases. A formal analogy of the Fokker–Planck equation with the Schrödinger equation allows the use of advanced operator techniques known from quantum mechanics for its solution in a number of cases. In many applications, one is only interested in the steady-state probability distribution , which can be found from . The computation of mean first passage times and splitting probabilities can be reduced to the solution of an ordinary differential equation which is intimately related to the Fokker–Planck equation.
Particular cases with known solution and inversion
In mathematical finance for volatility smile modeling of options via local volatility, one has the problem of deriving a diffusion coefficient consistent with a probability density obtained from market option quotes. The problem is therefore an inversion of the Fokker Planck–equation: Given the density f(x,t) of the option underlying X deduced from the option market, one aims at finding the local volatility consistent with f. This is an inverse problem that has been solved in general by Dupire (1994, 1997) with a non-parametric solution. Brigo and Mercurio (2002, 2003) propose a solution in parametric form via a particular local volatility consistent with a solution of the Fokker–Planck equation given by a mixture model. More information is available also in Fengler (2008), Gatheral (2008) and Musiela and Rutkowski (2008).
Fokker–Planck equation and path integral
Every Fokker–Planck equation is equivalent to a path integral. The path integral formulation is an excellent starting point for the application of field theory methods.[7] This is used, for instance, in critical dynamics.
A derivation of the path integral is possible in the same way as in quantum mechanics, simply because the Fokker–Planck equation is formally equivalent to the Schrödinger equation. Here are the steps for a Fokker–Planck equation with one variable x. Write the FP equation in the form
The x-derivatives here only act on the -function, not on . Integrate over a time interval ,
Insert the Fourier integral
This equation expresses as functional of . Iterating times and performing the limit gives a path integral with Lagrangian
The variables conjugate to are called "response variables".[8]
Although formally equivalent, different problems may be solved more easily in the Fokker–Planck equation or the path integral formulation. The equilibrium distribution for instance may be obtained more directly from the Fokker–Planck equation.
See also
- Kolmogorov backward equation
- Boltzmann equation
- Navier–Stokes equations
- Vlasov equation
- Master equation
- Bogoliubov–Born–Green–Kirkwood–Yvon hierarchy of equations
- Ornstein–Uhlenbeck process
- Cointelation SDE
Notes and references
43 year old Petroleum Engineer Harry from Deep River, usually spends time with hobbies and interests like renting movies, property developers in singapore new condominium and vehicle racing. Constantly enjoys going to destinations like Camino Real de Tierra Adentro.
Further reading
- Bruno Dupire (1994) Pricing with a Smile. Risk Magazine, January, 18–20.
- Bruno Dupire (1997) Pricing and Hedging with Smiles. Mathematics of Derivative Securities. Edited by M.A.H. Dempster and S.R. Pliska, Cambridge University Press, Cambridge, 103–111. ISBN 0-521-58424-8.
- Template:Cite doi
- Template:Cite doi
- Fengler, M. R. (2008). Semiparametric Modeling of Implied Volatility, 2005, Springer Verlag, ISBN 978-3-540-26234-3
- Crispin Gardiner (2009), "Stochastic Methods", 4th edition, Springer, ISBN 978-3-540-70712-7.
- Jim Gatheral (2008). The Volatility Surface. Wiley and Sons, ISBN 978-0-471-79251-2.
- Marek Musiela, Marek Rutkowski. Martingale Methods in Financial Modelling, 2008, 2nd Edition, Springer-Verlag, ISBN 978-3-540-20966-9.
- Hannes Risken, "The Fokker–Planck Equation: Methods of Solutions and Applications", 2nd edition, Springer Series in Synergetics, Springer, ISBN 3-540-61530-X.
External links
- ↑ 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534 - ↑ A. D. Fokker, Die mittlere Energie rotierender elektrischer Dipole im Strahlungsfeld, Ann. Phys. 348 (4. Folge 43), 810–820 (1914).
- ↑ M. Planck, Sitz.ber. Preuß. Akad. (1917).
- ↑ Andrei Kolmogorov, "On Analytical Methods in the Theory of Probability", 448-451, (1931), (in German).
- ↑ N. N. Bogolyubov (jr) and D. P. Sankovich (1994). "N. N. Bogolyubov and statistical mechanics". Russian Math. Surveys 49(5): 19—49.
- ↑ N. N. Bogoliubov and N. M. Krylov (1939). Fokker–Planck equations generated in perturbation theory by a method based on the spectral properties of a perturbed Hamiltonian. Zapiski Kafedry Fiziki Akademii Nauk Ukrainian SSR 4: 81–157 (in Ukrainian).
- ↑ 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534 - ↑ One of the biggest reasons investing in a Singapore new launch is an effective things is as a result of it is doable to be lent massive quantities of money at very low interest rates that you should utilize to purchase it. Then, if property values continue to go up, then you'll get a really high return on funding (ROI). Simply make sure you purchase one of the higher properties, reminiscent of the ones at Fernvale the Riverbank or any Singapore landed property Get Earnings by means of Renting
In its statement, the singapore property listing - website link, government claimed that the majority citizens buying their first residence won't be hurt by the new measures. Some concessions can even be prolonged to chose teams of consumers, similar to married couples with a minimum of one Singaporean partner who are purchasing their second property so long as they intend to promote their first residential property. Lower the LTV limit on housing loans granted by monetary establishments regulated by MAS from 70% to 60% for property purchasers who are individuals with a number of outstanding housing loans on the time of the brand new housing purchase. Singapore Property Measures - 30 August 2010 The most popular seek for the number of bedrooms in Singapore is 4, followed by 2 and three. Lush Acres EC @ Sengkang
Discover out more about real estate funding in the area, together with info on international funding incentives and property possession. Many Singaporeans have been investing in property across the causeway in recent years, attracted by comparatively low prices. However, those who need to exit their investments quickly are likely to face significant challenges when trying to sell their property – and could finally be stuck with a property they can't sell. Career improvement programmes, in-house valuation, auctions and administrative help, venture advertising and marketing, skilled talks and traisning are continuously planned for the sales associates to help them obtain better outcomes for his or her shoppers while at Knight Frank Singapore. No change Present Rules
Extending the tax exemption would help. The exemption, which may be as a lot as $2 million per family, covers individuals who negotiate a principal reduction on their existing mortgage, sell their house short (i.e., for lower than the excellent loans), or take part in a foreclosure course of. An extension of theexemption would seem like a common-sense means to assist stabilize the housing market, but the political turmoil around the fiscal-cliff negotiations means widespread sense could not win out. Home Minority Chief Nancy Pelosi (D-Calif.) believes that the mortgage relief provision will be on the table during the grand-cut price talks, in response to communications director Nadeam Elshami. Buying or promoting of blue mild bulbs is unlawful.
A vendor's stamp duty has been launched on industrial property for the primary time, at rates ranging from 5 per cent to 15 per cent. The Authorities might be trying to reassure the market that they aren't in opposition to foreigners and PRs investing in Singapore's property market. They imposed these measures because of extenuating components available in the market." The sale of new dual-key EC models will even be restricted to multi-generational households only. The models have two separate entrances, permitting grandparents, for example, to dwell separately. The vendor's stamp obligation takes effect right this moment and applies to industrial property and plots which might be offered inside three years of the date of buy. JLL named Best Performing Property Brand for second year running
The data offered is for normal info purposes only and isn't supposed to be personalised investment or monetary advice. Motley Fool Singapore contributor Stanley Lim would not personal shares in any corporations talked about. Singapore private home costs increased by 1.eight% within the fourth quarter of 2012, up from 0.6% within the earlier quarter. Resale prices of government-built HDB residences which are usually bought by Singaporeans, elevated by 2.5%, quarter on quarter, the quickest acquire in five quarters. And industrial property, prices are actually double the levels of three years ago. No withholding tax in the event you sell your property. All your local information regarding vital HDB policies, condominium launches, land growth, commercial property and more
There are various methods to go about discovering the precise property. Some local newspapers (together with the Straits Instances ) have categorised property sections and many local property brokers have websites. Now there are some specifics to consider when buying a 'new launch' rental. Intended use of the unit Every sale begins with 10 p.c low cost for finish of season sale; changes to 20 % discount storewide; follows by additional reduction of fiftyand ends with last discount of 70 % or extra. Typically there is even a warehouse sale or transferring out sale with huge mark-down of costs for stock clearance. Deborah Regulation from Expat Realtor shares her property market update, plus prime rental residences and houses at the moment available to lease Esparina EC @ Sengkang