Avogadro's law: Difference between revisions
en>Northamerica1000 m Reverted edit(s) by 24.131.171.12 identified as vandalism using STiki |
en>Myasuda m sp |
||
| Line 1: | Line 1: | ||
[[File:Symmetric_group_3;_Cayley_table;_matrices.svg|thumb|320px|Matrices describing the permutations of 3 elements<br> The [[Matrix multiplication|product]] of two permutation matrices is a permutation matrix as well.<br><br>These are the positions of the six matrices:<br>[[File:Symmetric_group_3;_Cayley_table;_positions.svg|310px]]<br>(They are also permutation matrices.)]] | |||
In [[mathematics]], in [[Matrix (mathematics)|matrix theory]], a '''permutation matrix''' is a square [[binary matrix]] that has exactly one entry 1 in each row and each column and 0s elsewhere. Each such matrix represents a specific [[permutation]] of m elements and, when used to multiply another matrix, can produce that permutation in the rows or columns of the other matrix. | |||
== Definition == | |||
Given a permutation π of ''m'' elements, | |||
:<math>\pi : \lbrace 1, \ldots, m \rbrace \to \lbrace 1, \ldots, m \rbrace</math> | |||
given in two-line form by | |||
:<math>\begin{pmatrix} 1 & 2 & \cdots & m \\ \pi(1) & \pi(2) & \cdots & \pi(m) \end{pmatrix},</math> | |||
its permutation matrix is the ''m × m'' matrix ''P''<sub>π</sub> whose entries are all 0 except that in row ''i'', the entry π(''i'') equals 1. We may write | |||
:<math>P_\pi = \begin{bmatrix} \mathbf e_{\pi(1)} \\ \mathbf e_{\pi(2)} \\ \vdots \\ \mathbf e_{\pi(m)} \end{bmatrix},</math> | |||
where <math>\mathbf e_j</math> denotes a row vector of length ''m'' with 1 in the ''j''th position and 0 in every other position.<ref name=Bru2>Brualdi (2006) p.2</ref> | |||
== Properties == | |||
Given two permutations π and σ of ''m'' elements and the corresponding permutation matrices ''P''<sub>π</sub> and ''P''<sub>σ</sub> | |||
:<math>P_{\sigma} P_{\pi} = P_{\pi\,\circ\,\sigma} </math> | |||
This somewhat unfortunate rule is a consequence of the definitions of multiplication of permutations (composition of bijections) and of matrices, and of the choice of using the vectors <math>\mathbf{e}_{\pi(i)}</math> as rows of the permutation matrix; if one had used columns instead then the product above would have been equal to <math>P_{\sigma\,\circ\,\pi}</math> with the permutations in their original order. | |||
As permutation matrices are [[orthogonal matrix|orthogonal matrices]] (i.e., <math>P_{\pi}P_{\pi}^{T} = I</math>), the inverse matrix exists and can be written as | |||
:<math>P_{\pi}^{-1} = P_{\pi^{-1}} = P_{\pi}^{T}.</math> | |||
Multiplying <math>P_{\pi}</math> times a [[column vector]] '''g''' will permute the rows of the vector: | |||
:<math>P_\pi \mathbf{g} | |||
= | |||
\begin{bmatrix} | |||
\mathbf{e}_{\pi(1)} \\ | |||
\mathbf{e}_{\pi(2)} \\ | |||
\vdots \\ | |||
\mathbf{e}_{\pi(n)} | |||
\end{bmatrix} | |||
\begin{bmatrix} | |||
g_1 \\ | |||
g_2 \\ | |||
\vdots \\ | |||
g_n | |||
\end{bmatrix} | |||
= | |||
\begin{bmatrix} | |||
g_{\pi(1)} \\ | |||
g_{\pi(2)} \\ | |||
\vdots \\ | |||
g_{\pi(n)} | |||
\end{bmatrix}. | |||
</math> | |||
Now applying <math>P_\sigma</math> after applying <math>P_\pi</math> gives the same result as applying <math>P_{\pi\circ\sigma}</math> directly, in accordance with the above multiplication rule: call <math>P_\pi\mathbf{g} = \mathbf{g}'</math>, in other words | |||
:<math>g'_i=g_{\pi(i)}\,</math> | |||
for all ''i'', then | |||
:<math>P_\sigma(P_\pi(\mathbf{g})) = P_\sigma(\mathbf{g}') | |||
= | |||
\begin{bmatrix} | |||
g'_{\sigma(1)} \\ | |||
g'_{\sigma(2)} \\ | |||
\vdots \\ | |||
g'_{\sigma(n)} | |||
\end{bmatrix} | |||
= | |||
\begin{bmatrix} | |||
g_{\pi(\sigma(1))} \\ | |||
g_{\pi(\sigma(2))} \\ | |||
\vdots \\ | |||
g_{\pi(\sigma(n))} | |||
\end{bmatrix}. | |||
</math> | |||
Multiplying a [[row vector]] '''h''' times <math>P_{\pi}</math> will permute the columns of the vector by the inverse of <math>P_{\pi}</math>: | |||
:<math>\mathbf{h}P_\pi | |||
= | |||
\begin{bmatrix} h_1 \; h_2 \; \dots \; h_n \end{bmatrix} | |||
\begin{bmatrix} | |||
\mathbf{e}_{\pi(1)} \\ | |||
\mathbf{e}_{\pi(2)} \\ | |||
\vdots \\ | |||
\mathbf{e}_{\pi(n)} | |||
\end{bmatrix} | |||
= | |||
\begin{bmatrix} h_{\pi^{-1}(1)} \; h_{\pi^{-1}(2)} \; \dots \; h_{\pi^{-1}(n)} \end{bmatrix} | |||
</math> | |||
Again it can be checked that <math>(\mathbf{h}P_\sigma)P_\pi = \mathbf{h}P_{\pi\circ\sigma}</math>. | |||
== Notes == | |||
Let ''S<sub>n</sub>'' denote the [[symmetric group]], or group of permutations, on {1,2,...,''n''}. Since there are ''n''! permutations, there are ''n''! permutation matrices. By the formulas above, the ''n'' × ''n'' permutation matrices form a [[Group (mathematics)|group]] under matrix multiplication with the identity matrix as the [[identity element]]. | |||
If (1) denotes the identity permutation, then ''P''<sub>(1)</sub> is the [[identity matrix]]. | |||
One can view the permutation matrix of a permutation σ as the permutation σ of the columns of the identity matrix ''I'', or as the permutation σ<sup>−1</sup> of the rows of ''I''. | |||
A permutation matrix is a [[doubly stochastic matrix]]. The [[Birkhoff–von Neumann theorem]] says that every doubly stochastic real matrix is a [[convex combination]] of permutation matrices of the same order and the permutation matrices are precisely the [[extreme point]]s of the set of doubly stochastic matrices. That is, the [[Birkhoff polytope]], the set of doubly stochastic matrices, is the [[convex hull]] of the set of permutation matrices.<ref name=Bru19>Brualdi (2006) p.19</ref> | |||
The product ''PM'', premultiplying a matrix ''M'' by a permutation matrix ''P'', permutes the rows of ''M''; row ''i'' moves to row π(''i''). Likewise, ''MP'' permutes the columns of ''M''. | |||
The map ''S''<sub>''n''</sub> → A ⊂ GL(''n'', '''Z'''<sub>2</sub>) is a [[faithful representation]]. Thus, |A| = ''n''!. | |||
The [[Trace (linear algebra)|trace]] of a permutation matrix is the number of fixed points of the permutation. If the permutation has fixed points, so it can be written in cycle form as π = (''a''<sub>1</sub>)(''a''<sub>2</sub>)...(''a''<sub>''k''</sub>)σ where σ has no fixed points, then '''''e'''''<sub>''a''<sub>1</sub></sub>,'''''e'''''<sub>''a''<sub>2</sub></sub>,...,'''''e'''''<sub>''a''<sub>''k''</sub></sub> are [[eigenvector]]s of the permutation matrix. | |||
From [[group theory]] we know that any permutation may be written as a product of [[transposition (mathematics)|transposition]]s. Therefore, any permutation matrix ''P'' factors as a product of row-interchanging [[elementary matrix|elementary matrices]], each having determinant −1. Thus the determinant of a permutation matrix ''P'' is just the [[signature of a permutation|signature]] of the corresponding permutation. | |||
== Examples == | |||
===Permutation of rows and columns=== | |||
When a permutation matrix ''P'' is multiplied with a matrix ''M'' from the left ''PM'' it will permute the rows of ''M'' (here the elements of a column vector),<br>when ''P'' is multiplied with ''M'' from the right ''MP'' it will permute the columns of ''M'' (here the elements of a row vector): | |||
{| style="text-align: center; width: 100%;" | |||
|style="width:50%"|[[File:Permutation matrix; P * column.svg|thumb|center|180px|''P'' * (1,2,3,4)<sup>T</sup> = (4,1,3,2)<sup>T</sup>]] | |||
|style="width:50%"|[[File:Permutation matrix; row * P.svg|thumb|center|257px|(1,2,3,4) * ''P'' = (2,4,3,1)]] | |||
|} | |||
Permutations of rows and columns are for example reflections (see below) and cyclic permutations (see [[Circulant matrix#Properties|cyclic permutation matrix]]). | |||
{| class="collapsible collapsed" style="width: 100%; border: 1px solid #aaaaaa;" | |||
! bgcolor="#ccccff"|reflections | |||
|- | |||
| | |||
{| style="text-align: center; width: 100%;" | |||
|style="width:50%"|[[File:Permutation matrix; row * P^T.svg|thumb|center|257px|(1,2,3,4) * ''P''<sup>T</sup> = (4,1,3,2)]] | |||
|style="width:50%"|[[File:Permutation matrix; P^T * column.svg|thumb|center|180px|''P''<sup>T</sup> * (1,2,3,4)<sup>T</sup> = (2,4,3,1)<sup>T</sup>]] | |||
|} | |||
These arrangements of matrices are reflections of those directly above.<br> | |||
This follows from the rule <math>\left( \mathbf{A B} \right) ^\mathrm{T} = \mathbf{B}^\mathrm{T} \mathbf{A}^\mathrm{T} \,</math> (Compare: [[Transpose#Properties|Transpose]]) | |||
|} | |||
===Permutation of rows=== | |||
The permutation matrix ''P''<sub>π</sub> corresponding to the permutation :<math>\pi=\begin{pmatrix} 1 & 2 & 3 & 4 & 5 \\ 1 & 4 & 2 & 5 & 3 \end{pmatrix},</math> is | |||
:<math>P_\pi | |||
= | |||
\begin{bmatrix} | |||
\mathbf{e}_{\pi(1)} \\ | |||
\mathbf{e}_{\pi(2)} \\ | |||
\mathbf{e}_{\pi(3)} \\ | |||
\mathbf{e}_{\pi(4)} \\ | |||
\mathbf{e}_{\pi(5)} | |||
\end{bmatrix} | |||
= | |||
\begin{bmatrix} | |||
\mathbf{e}_{1} \\ | |||
\mathbf{e}_{4} \\ | |||
\mathbf{e}_{2} \\ | |||
\mathbf{e}_{5} \\ | |||
\mathbf{e}_{3} | |||
\end{bmatrix} | |||
= | |||
\begin{bmatrix} | |||
1 & 0 & 0 & 0 & 0 \\ | |||
0 & 0 & 0 & 1 & 0 \\ | |||
0 & 1 & 0 & 0 & 0 \\ | |||
0 & 0 & 0 & 0 & 1 \\ | |||
0 & 0 & 1 & 0 & 0 | |||
\end{bmatrix}. | |||
</math> | |||
Given a vector '''g''', | |||
:<math>P_\pi \mathbf{g} | |||
= | |||
\begin{bmatrix} | |||
\mathbf{e}_{\pi(1)} \\ | |||
\mathbf{e}_{\pi(2)} \\ | |||
\mathbf{e}_{\pi(3)} \\ | |||
\mathbf{e}_{\pi(4)} \\ | |||
\mathbf{e}_{\pi(5)} | |||
\end{bmatrix} | |||
\begin{bmatrix} | |||
g_1 \\ | |||
g_2 \\ | |||
g_3 \\ | |||
g_4 \\ | |||
g_5 | |||
\end{bmatrix} | |||
= | |||
\begin{bmatrix} | |||
g_1 \\ | |||
g_4 \\ | |||
g_2 \\ | |||
g_5 \\ | |||
g_3 | |||
\end{bmatrix}. | |||
</math> | |||
== Explanation == | |||
A permutation matrix will always be in the form | |||
:<math>\begin{bmatrix} | |||
\mathbf{e}_{a_1} \\ | |||
\mathbf{e}_{a_2} \\ | |||
\vdots \\ | |||
\mathbf{e}_{a_j} \\ | |||
\end{bmatrix}</math> | |||
where '''e'''<sub>''a''<sub>''i''</sub></sub> represents the ''i''th basis vector (as a row) for '''R'''<sup>''j''</sup>, and where | |||
:<math>\begin{bmatrix} | |||
1 & 2 & \ldots & j \\ | |||
a_1 & a_2 & \ldots & a_j\end{bmatrix}</math> | |||
is the [[permutation]] form of the permutation matrix. | |||
Now, in performing matrix multiplication, one essentially forms the dot product of each row of the first matrix with each column of the second. In this instance, we will be forming the dot product of each row of this matrix with the vector of elements we want to permute. That is, for example, '''v'''= (''g''<sub>0</sub>,...,''g''<sub>5</sub>)<sup>T</sup>, | |||
:'''e'''<sub>''a''<sub>''i''</sub></sub>·'''v'''=''g''<sub>''a''<sub>''i''</sub></sub> | |||
So, the product of the permutation matrix with the vector '''v''' above, | |||
will be a vector in the form (''g''<sub>''a''<sub>1</sub></sub>, ''g''<sub>''a''<sub>2</sub></sub>, ..., ''g''<sub>''a''<sub>''j''</sub></sub>), and that this then is a permutation of '''v''' since we have said that the permutation form is | |||
:<math>\begin{pmatrix} | |||
1 & 2 & \ldots & j \\ | |||
a_1 & a_2 & \ldots & a_j\end{pmatrix}.</math> | |||
So, permutation matrices do indeed permute the order of elements in vectors multiplied with them. | |||
== See also == | |||
* [[Alternating sign matrix]] | |||
* [[Generalized permutation matrix]] | |||
==References== | |||
{{reflist}} | |||
* {{cite book | last=Brualdi | first=Richard A. | title=Combinatorial matrix classes | series=Encyclopedia of Mathematics and Its Applications | volume=108 | location=Cambridge | publisher=[[Cambridge University Press]] | year=2006 | isbn=0-521-86565-4 | zbl=1106.05001 }} | |||
[[Category:Matrices]] | |||
[[Category:Permutations]] | |||
[[Category:Sparse matrices]] | |||
Revision as of 16:14, 17 December 2013

The product of two permutation matrices is a permutation matrix as well.
These are the positions of the six matrices:
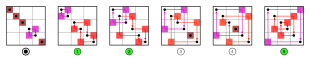
(They are also permutation matrices.)
In mathematics, in matrix theory, a permutation matrix is a square binary matrix that has exactly one entry 1 in each row and each column and 0s elsewhere. Each such matrix represents a specific permutation of m elements and, when used to multiply another matrix, can produce that permutation in the rows or columns of the other matrix.
Definition
Given a permutation π of m elements,
given in two-line form by
its permutation matrix is the m × m matrix Pπ whose entries are all 0 except that in row i, the entry π(i) equals 1. We may write
where denotes a row vector of length m with 1 in the jth position and 0 in every other position.[1]
Properties
Given two permutations π and σ of m elements and the corresponding permutation matrices Pπ and Pσ
This somewhat unfortunate rule is a consequence of the definitions of multiplication of permutations (composition of bijections) and of matrices, and of the choice of using the vectors as rows of the permutation matrix; if one had used columns instead then the product above would have been equal to with the permutations in their original order.
As permutation matrices are orthogonal matrices (i.e., ), the inverse matrix exists and can be written as
Multiplying times a column vector g will permute the rows of the vector:
Now applying after applying gives the same result as applying directly, in accordance with the above multiplication rule: call , in other words
for all i, then
Multiplying a row vector h times will permute the columns of the vector by the inverse of :
Again it can be checked that .
Notes
Let Sn denote the symmetric group, or group of permutations, on {1,2,...,n}. Since there are n! permutations, there are n! permutation matrices. By the formulas above, the n × n permutation matrices form a group under matrix multiplication with the identity matrix as the identity element.
If (1) denotes the identity permutation, then P(1) is the identity matrix.
One can view the permutation matrix of a permutation σ as the permutation σ of the columns of the identity matrix I, or as the permutation σ−1 of the rows of I.
A permutation matrix is a doubly stochastic matrix. The Birkhoff–von Neumann theorem says that every doubly stochastic real matrix is a convex combination of permutation matrices of the same order and the permutation matrices are precisely the extreme points of the set of doubly stochastic matrices. That is, the Birkhoff polytope, the set of doubly stochastic matrices, is the convex hull of the set of permutation matrices.[2]
The product PM, premultiplying a matrix M by a permutation matrix P, permutes the rows of M; row i moves to row π(i). Likewise, MP permutes the columns of M.
The map Sn → A ⊂ GL(n, Z2) is a faithful representation. Thus, |A| = n!.
The trace of a permutation matrix is the number of fixed points of the permutation. If the permutation has fixed points, so it can be written in cycle form as π = (a1)(a2)...(ak)σ where σ has no fixed points, then ea1,ea2,...,eak are eigenvectors of the permutation matrix.
From group theory we know that any permutation may be written as a product of transpositions. Therefore, any permutation matrix P factors as a product of row-interchanging elementary matrices, each having determinant −1. Thus the determinant of a permutation matrix P is just the signature of the corresponding permutation.
Examples
Permutation of rows and columns
When a permutation matrix P is multiplied with a matrix M from the left PM it will permute the rows of M (here the elements of a column vector),
when P is multiplied with M from the right MP it will permute the columns of M (here the elements of a row vector):
 |
 |
Permutations of rows and columns are for example reflections (see below) and cyclic permutations (see cyclic permutation matrix).
| reflections | ||
|---|---|---|
These arrangements of matrices are reflections of those directly above. |
Permutation of rows
The permutation matrix Pπ corresponding to the permutation : is
Given a vector g,
Explanation
A permutation matrix will always be in the form
where eai represents the ith basis vector (as a row) for Rj, and where
is the permutation form of the permutation matrix.
Now, in performing matrix multiplication, one essentially forms the dot product of each row of the first matrix with each column of the second. In this instance, we will be forming the dot product of each row of this matrix with the vector of elements we want to permute. That is, for example, v= (g0,...,g5)T,
- eai·v=gai
So, the product of the permutation matrix with the vector v above, will be a vector in the form (ga1, ga2, ..., gaj), and that this then is a permutation of v since we have said that the permutation form is
So, permutation matrices do indeed permute the order of elements in vectors multiplied with them.
See also
References
43 year old Petroleum Engineer Harry from Deep River, usually spends time with hobbies and interests like renting movies, property developers in singapore new condominium and vehicle racing. Constantly enjoys going to destinations like Camino Real de Tierra Adentro.
- 20 year-old Real Estate Agent Rusty from Saint-Paul, has hobbies and interests which includes monopoly, property developers in singapore and poker. Will soon undertake a contiki trip that may include going to the Lower Valley of the Omo.
My blog: http://www.primaboinca.com/view_profile.php?userid=5889534


























